These are standard hand rankings for most poker games and apply to all high-hand poker variations including Texas Hold'em, Omaha and Stud. You'll find a printable poker hand rankings chart below the hand rankings as well as answers to some of the most frequently asked questions about poker hands and poker hand ranking. List Of Poker Hands Best To Worst, kudos gambling game, mat roulettes, sands casino bethlehem food court. The table includes all cards’ combinations in the game arranged from the best in the top to the worst in the bottom. Royal Flush, Straight Flush, Four of a Kind, Full House, Flush, Straight, Three of a Kind, Two Pairs and Pair - all hands are represented in this table. Print out this free poker hand rankings chart – and always know the best winning poker hands. Poker hands are ranked in order from best to worst. Royal Flush An ace high straight flush. Straight Flush Five consecutive cards in the same suit. Four of a Kind Four cards of the same rank. 5-9 Not the worst of the 10 worst starting hands, but not one to get really happy about, either. 4-7, 4-8, 5-8, 3-6 These cards fall into the toss-em category, and unless you are in the big blind and can see the flop for free, let it go.
- Worst Starting Poker Hands
- What Are The Best Hands In Poker
- List Of Poker Hands From Best To Worst Dressed
This post works with 5-card Poker hands drawn from a standard deck of 52 cards. The discussion is mostly mathematical, using the Poker hands to illustrate counting techniques and calculation of probabilities
Working with poker hands is an excellent way to illustrate the counting techniques covered previously in this blog – multiplication principle, permutation and combination (also covered here). There are 2,598,960 many possible 5-card Poker hands. Thus the probability of obtaining any one specific hand is 1 in 2,598,960 (roughly 1 in 2.6 million). The probability of obtaining a given type of hands (e.g. three of a kind) is the number of possible hands for that type over 2,598,960. Thus this is primarily a counting exercise.
___________________________________________________________________________
Preliminary Calculation

Usually the order in which the cards are dealt is not important (except in the case of stud poker). Thus the following three examples point to the same poker hand. The only difference is the order in which the cards are dealt.
These are the same hand. Order is not important.
The number of possible 5-card poker hands would then be the same as the number of 5-element subsets of 52 objects. The following is the total number of 5-card poker hands drawn from a standard deck of 52 cards.
The notation is called the binomial coefficient and is pronounced “n choose r”, which is identical to the number of -element subsets of a set with objects. Other notations for are , and . Many calculators have a function for . Of course the calculation can also be done by definition by first calculating factorials.
Thus the probability of obtaining a specific hand (say, 2, 6, 10, K, A, all diamond) would be 1 in 2,598,960. If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of all diamond cards? It is
This is definitely a very rare event (less than 0.05% chance of happening). The numerator 1,287 is the number of hands consisting of all diamond cards, which is obtained by the following calculation.
The reasoning for the above calculation is that to draw a 5-card hand consisting of all diamond, we are drawing 5 cards from the 13 diamond cards and drawing zero cards from the other 39 cards. Since (there is only one way to draw nothing), is the number of hands with all diamonds.
If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of cards in one suit? The probability of getting all 5 cards in another suit (say heart) would also be 1287/2598960. So we have the following derivation.
Thus getting a hand with all cards in one suit is 4 times more likely than getting one with all diamond, but is still a rare event (with about a 0.2% chance of happening). Some of the higher ranked poker hands are in one suit but with additional strict requirements. They will be further discussed below.
Another example. What is the probability of obtaining a hand that has 3 diamonds and 2 hearts? The answer is 22308/2598960 = 0.008583433. The number of “3 diamond, 2 heart” hands is calculated as follows:
One theme that emerges is that the multiplication principle is behind the numerator of a poker hand probability. For example, we can think of the process to get a 5-card hand with 3 diamonds and 2 hearts in three steps. The first is to draw 3 cards from the 13 diamond cards, the second is to draw 2 cards from the 13 heart cards, and the third is to draw zero from the remaining 26 cards. The third step can be omitted since the number of ways of choosing zero is 1. In any case, the number of possible ways to carry out that 2-step (or 3-step) process is to multiply all the possibilities together.
___________________________________________________________________________
The Poker Hands
Here’s a ranking chart of the Poker hands.
The chart lists the rankings with an example for each ranking. The examples are a good reminder of the definitions. The highest ranking of them all is the royal flush, which consists of 5 consecutive cards in one suit with the highest card being Ace. There is only one such hand in each suit. Thus the chance for getting a royal flush is 4 in 2,598,960.
Royal flush is a specific example of a straight flush, which consists of 5 consecutive cards in one suit. There are 10 such hands in one suit. So there are 40 hands for straight flush in total. A flush is a hand with 5 cards in the same suit but not in consecutive order (or not in sequence). Thus the requirement for flush is considerably more relaxed than a straight flush. A straight is like a straight flush in that the 5 cards are in sequence but the 5 cards in a straight are not of the same suit. For a more in depth discussion on Poker hands, see the Wikipedia entry on Poker hands.
The counting for some of these hands is done in the next section. The definition of the hands can be inferred from the above chart. For the sake of completeness, the following table lists out the definition.
Definitions of Poker Hands
| Poker Hand | Definition | |
|---|---|---|
| 1 | Royal Flush | A, K, Q, J, 10, all in the same suit |
| 2 | Straight Flush | Five consecutive cards, |
| all in the same suit | ||
| 3 | Four of a Kind | Four cards of the same rank, |
| one card of another rank | ||
| 4 | Full House | Three of a kind with a pair |
| 5 | Flush | Five cards of the same suit, |
| not in consecutive order | ||
| 6 | Straight | Five consecutive cards, |
| not of the same suit | ||
| 7 | Three of a Kind | Three cards of the same rank, |
| 2 cards of two other ranks | ||
| 8 | Two Pair | Two cards of the same rank, |
| two cards of another rank, | ||
| one card of a third rank | ||
| 9 | One Pair | Three cards of the same rank, |
| 3 cards of three other ranks | ||
| 10 | High Card | If no one has any of the above hands, |
| the player with the highest card wins |
___________________________________________________________________________
Counting Poker Hands
Straight Flush
Counting from A-K-Q-J-10, K-Q-J-10-9, Q-J-10-9-8, …, 6-5-4-3-2 to 5-4-3-2-A, there are 10 hands that are in sequence in a given suit. So there are 40 straight flush hands all together.

Four of a Kind
There is only one way to have a four of a kind for a given rank. The fifth card can be any one of the remaining 48 cards. Thus there are 48 possibilities of a four of a kind in one rank. Thus there are 13 x 48 = 624 many four of a kind in total.
Full House
Let’s fix two ranks, say 2 and 8. How many ways can we have three of 2 and two of 8? We are choosing 3 cards out of the four 2’s and choosing 2 cards out of the four 8’s. That would be = 4 x 6 = 24. But the two ranks can be other ranks too. How many ways can we pick two ranks out of 13? That would be 13 x 12 = 156. So the total number of possibilities for Full House is
Note that the multiplication principle is at work here. When we pick two ranks, the number of ways is 13 x 12 = 156. Why did we not use = 78?
Flush
There are = 1,287 possible hands with all cards in the same suit. Recall that there are only 10 straight flush on a given suit. Thus of all the 5-card hands with all cards in a given suit, there are 1,287-10 = 1,277 hands that are not straight flush. Thus the total number of flush hands is 4 x 1277 = 5,108.
Straight
There are 10 five-consecutive sequences in 13 cards (as shown in the explanation for straight flush in this section). In each such sequence, there are 4 choices for each card (one for each suit). Thus the number of 5-card hands with 5 cards in sequence is . Then we need to subtract the number of straight flushes (40) from this number. Thus the number of straight is 10240 – 10 = 10,200.
Three of a Kind
There are 13 ranks (from A, K, …, to 2). We choose one of them to have 3 cards in that rank and two other ranks to have one card in each of those ranks. The following derivation reflects all the choosing in this process.
Two Pair and One Pair
These two are left as exercises.

High Card
The count is the complement that makes up 2,598,960.
The following table gives the counts of all the poker hands. The probability is the fraction of the 2,598,960 hands that meet the requirement of the type of hands in question. Note that royal flush is not listed. This is because it is included in the count for straight flush. Royal flush is omitted so that he counts add up to 2,598,960.
Probabilities of Poker Hands
| Poker Hand | Count | Probability | |
|---|---|---|---|
| 2 | Straight Flush | 40 | 0.0000154 |
| 3 | Four of a Kind | 624 | 0.0002401 |
| 4 | Full House | 3,744 | 0.0014406 |
| 5 | Flush | 5,108 | 0.0019654 |
| 6 | Straight | 10,200 | 0.0039246 |
| 7 | Three of a Kind | 54,912 | 0.0211285 |
| 8 | Two Pair | 123,552 | 0.0475390 |
| 9 | One Pair | 1,098,240 | 0.4225690 |
| 10 | High Card | 1,302,540 | 0.5011774 |
| Total | 2,598,960 | 1.0000000 |
___________________________________________________________________________
2017 – Dan Ma
Knowing your poker hands is crucial to playing a solid game at the table, and regardless of whether you’re playing Texas Hold’em, or Omaha, you’ll always want to know the value of your hand. When you have this knowledge at your disposal, you’ll be able to make an informed decision on whether to raise, check, call, or fold your hand when it matters most.
In this post, we’re going to look at the winning poker hands structure of two different games; Texas Hold’em, and Omaha – and we’ll begin by taking a look at a poker hands chart, followed by the best and worst starting hands. We’ll also show you a list of poker hands to help you make the right decisions in your game.
Worst Starting Poker Hands
Texas Hold’em Winning Poker Hands Ranking
In this guide, you’ll see that there are a total of 10 hands in Texas Hold’em poker (or 9 if you don’t count ‘no pair’ as a hand), and we’ll detail these below. (The winning poker hands chart below shows a list of poker hands, ranked best, to worst).
Royal Flush: Ten, Jack, Queen, King, Ace, all of the same suit.
Straight Flush: Any 5 cards of the same suit, in consecutive order. (I.e. 5, 6, 7, 8, and 9 of spades).
4-of-a-kind: 4 cards of the same value (i.e. the 5 of spades, the 5 of hearts, the 5 of clubs, and the 5 of diamonds).
Full House: A full house consists of one 3-of-a-kind hand, and one pair, so for instance, a full house could be the 2 of spades, the 2 of diamonds, the 2 of clubs, and a pair of Aces.
Flush: Five cards of the same suit (i.e. 2, 3, 7, 8, and 9 of hearts).
Straight: Five cards in consecutive order (i.e. 2, 3, 4, 5, 6, off-suit).
3-of-a-kind: Three cards of the same value (I.e. 3 of clubs, 3 or spades, and the 3 of hearts).
Two Pair: Two pairs in one hand – i.e. a pair of 2’s, and a pair of 3’s.
One Pair: One singular pair – i.e. a pair of Aces.
No Pair: A no pair hand is when you don’t have any of the above. In this instance, you have what is known as a ‘high card hard’.
Use the poker hands chart above to ensure you’re always in full control of your game!
Omaha Poker Hands Ranking
Omaha uses the exact same hand-ranking process as Texas Hold’em does, and while it may seem as though that’s a little ‘odd’ at first, remember that Omaha is almost identical, aside from the fact that players have four cards, and that betting is usually pot-limit.
This means that a Royal Flush is the best possible hand in a game of Omaha, and high-card (while incredibly unusual due to the 4 cards each player holds) is the lowest possible hand.
What Are The Best Hands In Poker
For a full guide on playing Omaha, be sure to check out our How to Play Omaha guide.
Best Starting Poker Hands

Knowing your winning poker hands is crucial if you want to play a solid game of poker – and below, we share how to determine whether or not you have a winner on your hand!
Texas Hold’em: The best starting hand is a pair of Aces. A pair of aces (also known as pocket rockets) are a favourite pre-flop over any other starting hand and is almost always one of the best winning poker hands. The second, and third best starting hands are a pair of Kings and Queens respectively, followed by Ace-King suited, pocket Jacks, pocket Tens, and then Ace-Queen suited. The 10th best starting hand is Ace-King Offsuit – which is actually still a very strong hand.
Omaha: Working out winning poker hands in Omaha is a little more complex than Texas Hold’em, when looking at the best starting hands, although mathematically, the best starting hands can be determined.
List Of Poker Hands From Best To Worst Dressed
For example, the strongest hand is AAKK, followed by AAJT, AAQQ, and AAJJ. It’s worth noting however, that the best possible hand in Omaha holds little value against a full-ring of players, hence the need to play aggressively, pre-flop and post-flop; see our poker hands chart for more details.
Worst Poker Starting Hands
Determining the worst starting hands in poker is a little more challenging – largely due to the fact that most players simply have knowledge of the better starting hands – yet knowing what the worst starting hands are is just as important, as it allows you to know when to throw away your hands pre-flop. Below, we look at the worst starting hands for both Texas Hold’em and Omaha.
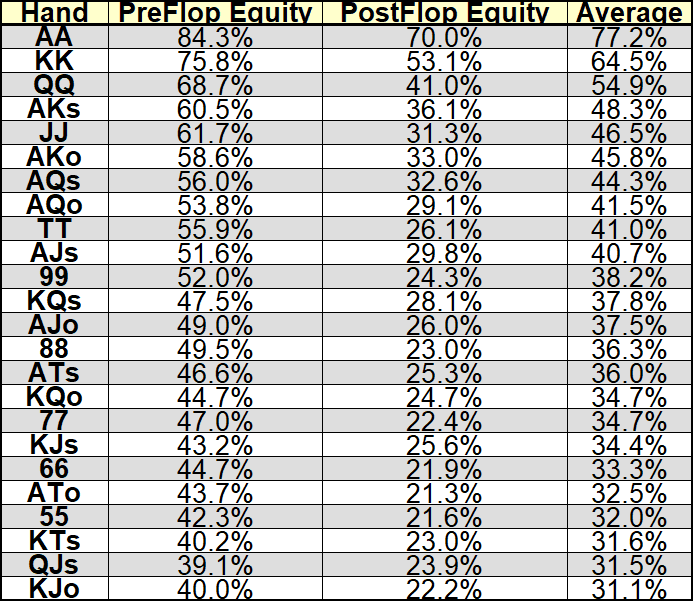
Texas Hold’em: 2-7 offsuit (this is well-known as the worst starting hand in poker, due to the fact that it’s often used as side-bets in games.) 7-2 offsuit is followed by 8-2 offsuit, 8-3 offsuit, 7-3 offsuit, 6-2 offsuit, 9-2, 9-3, and 9-4 offsuit.
Omaha: Unfortunately, with Omaha, there isn’t an ‘official’ list of worst poker hands – purely due to the fact that there would be too many to list. However, most professionals and poker experts agree that any starting hand that combines any of the worst starting hands in Texas Hold’em generally constitute a very poor starting hand in Omaha too – so it’s worth throwing these away most of the time, pre-flop. If in doubt, consult the list of winning poker hands ranking above.